Seismo Blog
How Earthquakes Break the Speed Limit
Categories: Indonesia | Earthquake Science | Earthquake Faults and Faulting
March 8, 2019
One of the reasons earthquakes are so dangerous is that they are fast, really fast. Consider seismic waves: Even the slowest common wave type, the Rayleigh waves, circles the globe with speeds of more than 7,000 miles per hour. The fastest P-waves plow through the Earth's interior at 18,000 miles per hour – roughly the same velocity with which the astronauts on board the International Space Station orbit our planet. Speed is also of the essence in the focus or the hypocenter of the earthquake itself. There rocks break fast and from there the rupture propagates at speeds faster than that of a jet in supersonic flight.
In contrast to common belief, earthquakes are not just the simple dots with which they are always depicted on a map. These dots – called epicenters – solely indicate the point on the Earth's surface under which the earthquake rupture process originates. This location is typically several miles below ground. Depending on the magnitude of the earthquake such ruptures can be dozens, if not hundreds of miles long. For a small temblor of magnitude 5 the rupture will typically extend only for a couple of miles. During the magnitude 7.9 San Francisco earthquake of 1906 for instance, the San Andreas Fault ruptured between east of Santa Cruz and its northern terminus at Cape Mendocino in Humboldt County, a distance of almost 300 miles. And in one of the strongest quakes ever measured, the magnitude 9.2 Great Sumatra earthquake of 2004, the rupture extended for more than 750 miles – almost the length of California.
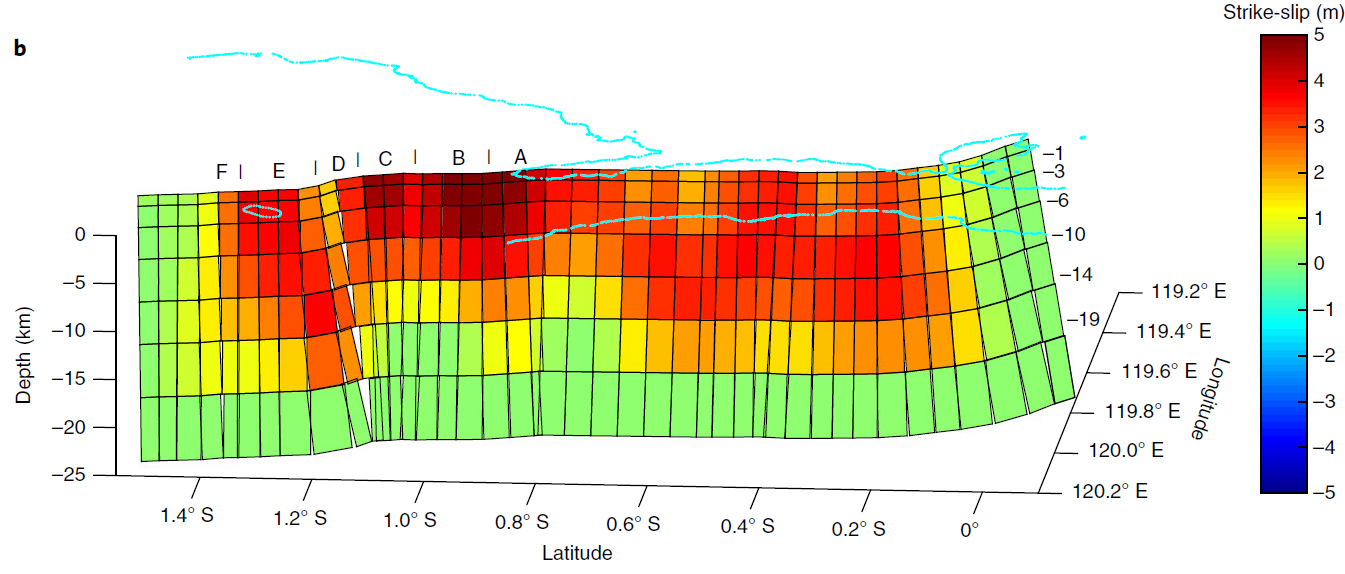
Figure 1: This vertical cut through the top 16 miles of the Earth's crust shows how much the ground shifted during the Palu Earthquake. The horizontal axis represents approximately 100 miles of the north-south extending Palu-Koro Fault, which ruptured during the quake. Areas in green show no slip, while the darkest brown colors depict areas with the largest slip of more than 15 feet. This is under the sections labeled A, B and C, where the fault cuts right through the city of Palu. In these sections the quake's rupture may have exceeded the theoretical "speed limit". Source: Socquet et al., Nature Geoscience
During a quake, the fault does not rupture all at once. Instead, starting from the hypocenter, the actual breakage of the bonds between the rocks on the opposing flanks of the fault propagates rapidly along the fault line. This process is similar to opening a zipper, which also does not pop open all at once. Instead the opening propagates along the lines of the interlocking teeth. In case of a zip fastener this propagation speed depends on the force with which the operator pulls on the slider. In an earthquake this so called rupture speed is controlled by the physical parameters of the rock involved. Typically, an earthquake rupture propagates somewhat slower than the velocity at which seismic waves travel, but still at impressive speeds about ten times the speed of sound in dry air. At such a speed, it will have taken more than a minute and a half for the rupture of the 1906 San Francisco quake to travel from its hypocenter off the Golden Gate all the way north to Cape Mendocino.
For the longest time seismologists have thought that there is a theoretical limit to the rupture velocity. Although some laboratory measurements at very high pressures and a handful of observations during actual earthquakes suggested higher velocities, about the speed of Rayleigh waves was considered the speed limit at which any rupture would propagate. That would translate to a maximum speed of approximately two miles a second or 7,200 miles per hour. But now evidence is mounting that earthquakes can indeed break this postulated speed limit.
Independently of each other, two research groups have found that during the magnitude 7.5 earthquake, which devastated the city of Palu on the Indonesian island of Sulawesi at the end of September last year, the rupture propagated faster than thought possible. Using teleseismic measurements Han Bao from the University of California, Los Angeles, and colleagues computed a rupture speed of almost three miles a second. Using satellite observations French researchers led by Anne Socquet from the University of Grenoble arrived at a similar conclusion.
How can this happen? Currently, there are two preliminary explanations. One reason is that the Palu-Koro Fault, which runs north-south through the densely populated city of more than 300,000 inhabitants and which ruptured for about 100 miles during the quake, is extremely straight. It has no bends or small jumps. Hence, like a car can travel much faster on a straight freeway than on a curvy highway in the country, the rupture may picked up its speed along the very straight fault line. Another possible cause for the high speed quake rupture may have to do with the friction along this straight section of the fault. The friction, or the resistance to rapid movement, seems to have been rather low, hence there was less loss of initial tectonic energy during the rupture propagation.
The causal observer may rightly ask, why does it matter how fast earthquake ruptures race through the country side. Isn't of purely academic interest to determine whether quakes break the speed limit? Well, not really, as the comparison to a real world car crash shows. If a car crashes into a wall at low speed, say ten miles an hour, the vehicle may suffer only superficial damage. On the other hand, a similar crash at 50 miles an hour may have deadly consequences and the car may be totaled. In the same way, fast earthquakes carry a stronger punch and hence more energy to wreak havoc on buildings and infrastructure. In addition, the faster a quake's rupture propagates, the less time there is for earthquake early warning – and in this business every second counts and can save lives. (hra163)
Both studies were recently published in "Nature Geoscience". Bao's group at doi: 10.1038/s41561-018-0297 and Socquet's team at doi: 10.1038/s41561-018-0296
BSL Blogging Team: Who we are
Recent Posts
-
: Alerts for the Whole West Coast
-
: Destruction in the Eastern Aegean Sea
-
: An Explosion in Beirut heard all over the Middle East
View Posts By Location
Categories
- Alaska (3)
- Bay Area (24)
- Buildings (3)
- Calaveras (4)
- California (13)
- Central California (4)
- Chile (4)
- Earthquake Early Warning (10)
- Earthquake Faults and Faulting (44)
- Earthquake Science (3)
- Haiti (3)
- Hayward (12)
- Indonesia (4)
- Induced Seismicity (3)
- Instrumentation (18)
- Italy (6)
- Japan (7)
- MOBB (3)
- Mendocino Triple Junction (5)
- Mexico (7)
- Nepal (3)
- North Korea (5)
- Nuclear Test (5)
- Ocean Bottom Seismometer (3)
- Oklahoma (4)
- Plate Tectonics (18)
- Preparedness, Risks, and Hazards (15)
- Salton Sea (3)
- San Andreas Fault (14)
- Seismic Waves (13)
- Seismograms (4)
- ShakeAlert (3)
- Southern California (5)
- Surface Waves (3)
- Today in Earthquake History (20)
- Volcanoes (4)
- subduction (3)